International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1374

ISSN 2229-5518

The Topological Property of Topological Manifold- Compactness with Cut Point and Punctured point.

Shri Haloli H.G.

ABSTRACT:-

We conduct a study on Topological manifold and one of its property compactness with cut point and punctured point. Some of theorems, structures on Topological manifold and its structural characteristics. The concepts like local and global property of manifold with some cut point or puncture point, local compactness, path in compact space, cut point in compact space and property on Topological manifold.

Index Terms :-

Boundedness, compactness, cut point, limit point, path space as metric space, punctured space.

1.INTRODUCTION:-

We are using some basic concepts like, limit point, cut point, boundedness, cover, compact space local property on Topological Manifold. The meaning of compactness in Topology is many concepts, the main abstraction of a concept from the real numbers, chronologically; it was first proved that subsets of R that are closed and bounded have a number of important properties. It was subsequently proven, in what is now called the Heine-Borel Theorem that if a subset E of R is closed and bounded, then it has the following property, E is contained in any union of infinitely many open sets, then, it is contained in the union of only finitely many of them. The conclusion of this theorem was subsequently made a definition called compactness; compact topological spaces exhibit many of the delightful properties of closed and bounded subsets of R.
Some of basic concept of compactness is depends on
cover, which are defined as follows:

Definition:1.1 [12]

Let X be a topological space and let E be a subset of X. a collection ϵ of open subsets of X is called an open cover of E if the union of all sets in C contains E.
A sub collection of an open cover that is itself a cover is called a sub cover. That is if E is subset of a topological space with open cover C and C’ subset of Cs a cover of E, then C’ is a sub cover for C-A sub cover is called a finite sub cover if it contains only finitely many open sets.

Definition 1.2:

A subset E of a Topological space X is compact if every open cover of E has a finite sub cover. A topological space X is itself compact if every open cover of X (by open sets in X) has a finite sub cover.

Theorem 1.3[12]

A closed subset of a compact space is compact.

International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1375

ISSN 2229-5518

A topological space E is said to be have the Bolzano-Weierstrass property if every infinite subset of E as a limit point in E.

Subset of E as a limit point in E.

We have every closed and bounded set E subset of R and every infinite subset of E has a limit point in E. This proved that every closed and bounded subset of R has the Bolzano-Weierstras property.

Theorem 1.4 (Bolzano- Weierstrass Theorem).

Every compact space has the Bolzano Weierstrass property.
In compact space the major application with
metric space. Now for topological manifold path is major connection between two point which are closed in compact space.
In the second section we redifined some basic and
required idefinations on Topological Manifold.
In the third section the main part of the paper . In this section we defined cut path on compact space and effect of cut path with the help of path connectedness defined by [6] .
Lastly we conclude the concept of cut point and
punchered space are effects on compact space and this concept we are using in removeal of cut point and punchered space with help of covering theorem which retains the compactness property of a topological space and makes the space stonger and stronger .
In the next section we defined some basic definition.

2. SOME BASIC DEFINITIONS:-

Definition:

2.1 Cut point:-

A point x in a Topological space is said to cut point if by removal of x, X becomes disconnected as
separation.

Definition 2.2

A strong cut point

A topological space X is called a strong cut point if the Topological space X contains only one cut point or cut path in X.
X – {x} is disconnected

Definition 2.3 Path connected.

A surface is a path connected if given any two points x ,yϵ S there is a map γ: [0,1] S such that γ (0) = x and γ (1)= y. The map γ is called path in S from x to y.

Definition 2.4

A path space (M, γ) is a set M equipped with a function γ :MxMR satisfying the following axis’s for x, y and z in M .
i) γxy ≥ 0, Ƴ xx ≥ 0 for x = y then Ƴ is a loop
ii)Ƴxy = Ƴ yx Symmetry path
iii) γXZ = γ XY + γ YZ lift of path.
The function γ isa path on M and γ XY is the path between x and y.
γXY is the path from x to y.
The collection of all paths in M is base for a topology on M. This topology on M induced by γ. This collection forms a net in M which closed and bounded by paths.
Then M is compact as path space.

Definition 2.5

Punctured point:

A point x in topological space X is called punctured point if X-(x) is connected.

Definition 2.6.

A topological space is called punctured space if it contains at east one punctured point.

International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1376

ISSN 2229-5518

Definition 2.7 Limit point compact :

A space X is said to be limit point compact if every infinite subset of X has a limit point in X and sequentially compact if every sequential (Sequences of points) in X has subsequence that converges to limit point.

Definition 2.8 Locally compact:

A topological space X is said to be locally compact if for every x belongs to X, there is a compact subset of X containing a neighboring of X.
Compactness Locally Compact

3. Compactness:-

Compactness is plays an important role in topological manifold. A space is compact it every open cover has a finite subcover,Robbery Bonnet and Matalyahu Rubin [10] introduce. The continuous images of compact ordered spaces. A topological space X is called a close open (CO) space if every closed Subset of X is homeomorphic to some
cl-open subset of X.

Definition 3.1 [10]

A compact Hausdorff space X is scattered if every non-empty subset A of X has an isolated point in its relative’s topology.
Let X be a Topological space X be ct x of X, i.e x be
cut point of X, if A⊆ X , B ⊆ X subset then A∪B=X,
A∩B=ctX.
If any xЄX, then set of open neighborhoods of x in X is denoted by NbrX((x) and the set of closed neighborhoods of X in X is denoted by Nbr X((x) – NbrX(x) clp The set of close-open neighborhoods of X in X.
As defined in previous paper path between A and B which passing through cut point of X. By removal of cut point ctx, the path becomes cut such path is defined as –

Definition 3.2 Cut path in X

Let X be a topological space, A and B are subset of X such that AUBЄ X, and A∩B=ctx, Ax and B y are two component of X for all x ЄA, y ЄB, except ctx point. A path γ(o)=x, γ(1)=y becomes a cut such path is called cut path in X.

Theorem 3.3 [7]

Compactness implies limit point compactness.

Lemma: 3.4 [7]

For first countable Hausdorff space limit point compactness implies sequential compactness.

Lemma 3.5 [7]

Suppose~: X Y is a quotient map and K is a locally compact Hausdorff space then map ~ X Id:
XxK YxK is a quotient map.

Definition 3.6 Punctured point:

Let X be compact space, x ЄX be any point X. The point x is to be punctured by removal of point x from X, X becomes compact.
i.e X be compact space than
X-{x} again compact, x is punctured point.

Theorem 3.7

A compact surface with finitely many holes is also compact.

International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1377

ISSN 2229-5518

Proof:

Let X be compact surface
Let {xi } be set of all punctured points of X.
A subset E of a Topological space X is compact
Therefore X is closed and bounded subsets of X.
By theorem 1.4, E has the Bolzano- Weierstrass property i.e every infinite sub set of E has a limit
point in E.
The Nbrc X(Xi)is closed point of X which are in X, which is compact space.
E be subset which contains finitely many hole. By punctured points definition E is still compact this prove that any compact surface with finitely many holes is also compact.

Remarks 3.8

A compact surface with infinitely many holes is non compacti.e non-compact space which infinitely many holes

Ex: Dossa :
A compact surface with finitely many holes

A compact surface with finitely many holes
Non compact surface with infinitely may hole.
The non compact surface repeats
infinitely in both direction.

Theorem 3.9 [12]

A closed subset of a compact space is compact.

Let X be a path space. Define of the diameter of a bonded subset A is a subset of X of the real number diameter (A)=supremum (γXY /x,yЄA) Suppose that C is an open cover of a metric space X. If there exists a
number 𝛿>0 such that any set of diameter less than
r/2 is contained in some open set O ϵ C then we call it

𝛿 a Lebesguenumber for open cover C

Theorem 3.10

Every open cover of a compact path space has a

Lebesgue number.

Proof:

Let X be a compact path space and let C be an open cover of X. Suppose to obtain a contradiction that C has no Lebesgue number. Then for every n Є N there exists a set An of diameter less than 1/n that is not
contained in any open set in C.

International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1378

ISSN 2229-5518

For each n, choose a point xn in An. If {xn } is a finite subset of X, then some point appears in the list x1 x2……. infinitely many times and we called this point x.
If {xn } is an infinite subset of X, than {xn } has a limit
If γ and 𝜎 are path in X such that the end point of γ is the beginning point of 𝜎 then we can combine γ and

𝜎 to form a new path that follows 𝜎 and then γ.

𝜎 (2S) if 0 ≤ S ≤ 1/2

point by the Bolzana Weierstrass theorem and we call this point x.

𝛾 𝑜 𝜎 ={

γ(2S-1) If 1/2< S ≤ 1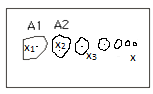

In either case, x is contained in some set O of the open cover. Since O is open there exists an r>0 such that Br(x) O By our choice of x there exists some KЄN such that L(γ(xxk ) < r/2 and diam (Ak ) <r/2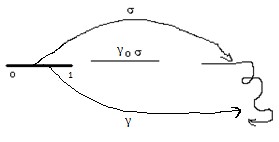

The path γ 0 𝜎R is called the product of γ and 𝜎
The product of the path 𝜎 with the path γ.
Let γ is path from x to y and 𝜎 is also from t to x.

𝜎

t x 𝛾 y

𝛾𝑜𝜎

Br/2 (. xk)

Br(x)

Theorem 3.12

Let X be compact topological space and (X, γ) is a

path space the following statement are hold.

1) There exists a path between any two point in X.

2) If {xi } are set of punctured point in X. The X is locally compact space.

Proof.

Then by the triangle inequality Ak is a subset of Br/2 (Xk ) is a subset of Br(x) is a subset of O.
This showed that a compact subset of R is closed.

Theorem 3.11 (12)

A compact subset of a Hausdorff space is closed.

By the composition of two path is defined by William
Basenar [12](P-220).
Let X be compact Topological space and (X ,γ) is a
path space (X γ) satisfies the condition that definition
2.4.
There exist path from x and y with condition of summitry also as X is compact each path in X has closed subset of path which maps in composition from which converges with limit point which also containing its neighborhood of X. this show each sub path is joined by sequence of such points in x.
This proves the statement (i).

International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1379

ISSN 2229-5518

(ii) statement proved by Theorem 3.7.
As X is compact surface with {xi } are punctures points (Holes) is also compact.
Every compact space is locally compact.

Corollany 3.13

Compactness implies sequentially compact.

Proof

By theorem 3.3 [7]
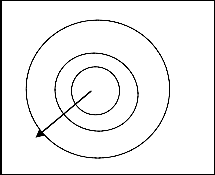
Compactness implies limit point compact also Lemma

3.4 limit point compactness implies sequential compactness.
Compact Limit point Compact

Sequential Compact

Locally Compact

Conclusion:-

In this we studied compactness property in
Topological Manifold. Effect of cut point and
punctured space on Topological Manifold. Main aim of
study cut point and punctured space, is that, I studied on Fiber bundle and by using path space, covering spaces, making topological space stronger and stronger, which is applying in fiber bundles.
------------------------------------------------------------------- Author’s, Shri Haloli H.G( Researcher)
Department Of Mathematics
Karnatak University , Dharwad
E- Mail: halolih@yahoo.co.in

Reference

[1].Antoni.a.kosinski(1993) “Differential Manifolds”
Academic press.inc
Harcourt Brace Jovanovich, Publisher
Boston sandiego New york
London Sydneytokyo Toronto
[2] B.Honan Y Bahrampour (1999) “ Cutpont space”
Proceedings of America Math Society 127 (p- 2797-2003)
[3] Devender.K.Kamboj and Vinodkumar (2010) “Cut point in some connected topological
spaces”
. Topology And its Application 157(2010)629-
634)
[4] Devender. K. Kamboj and Vinodkumar
(2009)
“H(i) connected topological spaces and
Cutpoints”
Topology And its application 156
p(610- 615)
[5] G.T.Whyburn(1928) “Concerning the cut point of
continue.”
Trans.Amer.Math.Soc.30(1928)597-609 [6] Haloli H.G.(2013)
“Conectedness and Punctured Space in Fiber bundle space ”
International journal of Engineering Research and Technology (IJERT),
ISSN -2278- 0181,Vol.-2 Issue 6 June-2013(p-2389-
2397)
[7] John.M.LEE(2004)
“Introdution to Topological Manifold” Springer.com(USA)
[8] MarcLackenby(2010)
“ Finite Covering Space of manifolds Proceedings of the International Congress of Mathematicians”
HyderbadIndia(p1042-1070)

International Journal of Scientific & Engineering Research, Volume 4, Issue 8, August-2013 1380

ISSN 2229-5518

[9] Pascal Collin ,Robert Kusner,William H meeks and Harold
“The topology Geometry and conormal structure of properly embedded minimal
surfaces”
J.Differential Geometry 67(p-377-393)
[10] Robert Bonnet and Matatyahu Rubin (2008) “A classification of continuous images of compact (co) space which are continuous images of compact Ordered space.”
J. Topology and its application155-(2008)
Elsevier-Science Direct p375-411 [11] William M. Boothby (2008)
“An introduction to Difererential Manifold and
Riemannian Geometry “
Academic press.
[12]. William F Basener (2006)
“Topology and its applications Wiley-Interscience.
A John Wiley & sons Inc.Publication