(3)
where i is the bit position of the coded bit in a constellation symbol, m is the number of information bits per symbol
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 1
ISSN 2229-5518
Abstract: Coded modulation is a bandwidth efficient schemes that combines the function of coding and modulation. In this paper a comparative study of various modulation techniques such as Trellis coded modulation(TCM),Turbo Trellis coded modulation(TTCM),Bit-Interleaved Coded Modulation(BICM)
,Iterative Decoding - Bit-Interleaved Coded Modulation(BICM-ID) and Superposistion Coded Modulation schemes over Gaussian and Rayleigh Fading
channels is made and presented in the context of 8 level PSK. The study is based on the comparison made for associated decoding complexity; bloc k length and bandwidth efficiency for static channel scenario. It is shown that SCM constitutes the best compromise scheme, followed by BICM-ID.
Index Terms : SCM –Superposistion Coded Modulation ,BER-Bit Error Rate, PAPR-Peak to average power ratio, OFDM-Orthogonal Frequency Division Modulation. BICM- ID -Bit Interleaved coded Modulation for Iterative Decoding. QAM- Quadrature Amplitude Modulation, QPSK- Quadrature Phase Shift Modulation..
………………………………………………………………………………………………………………………………………………………………………………….
In wireless communication efficient utilization of the bandwidth is the major issue due to the increasing demands from the users. In Coded modulation scheme both coding and modulation are combined to efficiently utilize the available bandwidth. There are many coded modulation schemes have been proposed in literature. In this paper we will briefly discuss the conventional coded modulation schemes in the present section .The conventional coded modulation are based on uniformly spaced constellation with equal probability. Trellis coded modulation proposed by Ungerbeoek performs well for Gaussian channels. Another coded modulation Turbo Trellis Coded Modulation proposed by Robertson employs turbo codes, but the difference is that TTCM requires 0.5dB lower SNR only at the BER of 10-4 than turbo coded schemes for 8PSK over AWGN channels.
Both TCM and TTCM employs set partitioning based signal labeling to achieve a high free Euclidian distance for AWGN. By incorporating random interleavers to the above coded schemes, we can reduce decoding complexity. BICM proposed by Zehavi utilizes bit-based interleavers in conjunction with Gray signal constellation labeling. It combines both convolution codes with bit interleavers. This scheme reduces free Euclidean space. The performance of BICM is better than TTCM and TCM over uncorrelated Rayleigh channels, but performs worse in
AWGN channels. For iterative decoding and demodulation
the BICM is modified, and modified BICM is referred as BICM-ID that is proposed by Li.Ping. This scheme uses signaling Partition labeling as in TCM and TTCM, but increases the BICM Free Euclidian distance space. Hence it exploits the full advantage of bit interleaving with the aid of soft-decision feedback based iterative decoding.
This paper is organized as follows: in Section 2 introduces the encoding and decoding techniques for conventional coded modulation systems. Section 3 introduces the basic system model contains an information- theoretic analysis of clipping effect. Section 4: Comparison of SCM with BICM is presented. Finally, we summarize our main results in section 5.
A random Information bits are generated and encoded by one of the TCM, TTCM, BICM and BICM encoders and resultant coded sequence is modulated by an appropriate modulation scheme and transmitted. We have considered as 1. Static channels. 2. A narrow band Rayleigh fading channel for comparison. With coherent detection, the relationship between the transmitted discrete time signal xt and the received discrete time signal yt is given by[9].
yt =ptxt + nt (1)
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 2
ISSN 2229-5518
where pt is the Rayleigh-distributed fading amplitude having an expected value of E[pt ]2 =1 ,pt .For AWGN pt=1, and nt is the complex AWGN with variance σI 2 = σQ 2 =No/2 we have considered the receiver as the coherent demodulator followed by a deinterleaver and one of the TCM, TTCM or BICM decoders. In case of BICM and BICM-ID schemes the decoder output is followed by appropriate deinterleaver, the output of the deinterleaver is fed back to the demodulator input.
The branch metric for TCM and TTCM for the maximum likelihood decoding over fading channels is
π t =|yt-ptxt|2 (2)
The corresponding branch metric for BICM and BICM-ID is formed by summing the deinterleaved bit metrics ƒ of each
coded bit vti , yielding
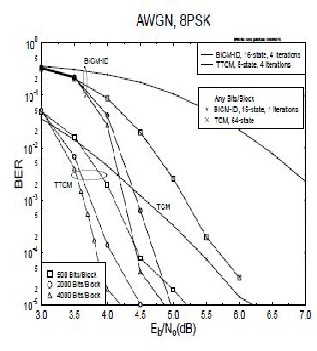
the figure that a high interleaving block length is necessary for the iterative TTCM and BICM schemes .But the block length does not have an influence on the BICM-ID performance during the first iteration. Since it constitutes a BICM scheme using SP based signal labeling. At a BER of
10-4 a 500 bit block length was about 1dB inferior in SNR terms in comparison to the 2000 bit block length in the context of the BICM-ID scheme.
t
m1
i 1
f V i b
(3)
where i is the bit position of the coded bit in a constellation symbol, m is the number of information bits per symbol
and b ò 0,1 . One parity bit is added to the m information
bits, due to the addition of the parity bits the original constellation size will increase. The BICM bit metrics 
before the deinterleaver are defined as [7]
Figure 1: Effects of block length on the TCM, TTCM and
f i
b
m
in 
yt pxt 
(4)
BICM-ID performance in the context of an 8PSK scheme over AWGN channels.
The coded modulation schemes that we comparatively studied are TCM proposed by Ungerboeck [2], TTCM proposed by Robertson [4], BICM proposed by Zehavi's [6] and BICM-ID proposed by Li. [8]. TCM and TTCM codes are in octal format. By the addition of one parity bit to the information the coding rate for a 2 m+1 - ary signal in PSK or QAM is given by R = m/m+1 and the number of decoding states for a code of memory K is 2K. .
In Figure.1 the performance of the TCM, TTCM and BICM-ID with respect to the block length with an 8PSK scheme over AWGN channels is depicted .It is clear from
The figure2 shows the performance of 64 states TCM and BICM and 8 states TTCM using four iterations and 16 states BICM-ID employing four iterations in an
8PSK scheme .As may be seen from the figure 2, TTCM performs best, followed by BICM ID, BICM, TTCM and TCM. At the BER of 10-4, TTCM performs about 0.7dB better than BICM-ID, 2.3dB better than BICM and 4.5dB better than TCM.
For a given complexity TCM performs better than BICM in AWGN channels, but worse in uncorrelated narrowband Rayleigh fading channels. However, BICM-ID using soft decision feedback outperforms TCM and BICM
over both AWGN and uncorrelated narrowband Rayleigh
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 3
ISSN 2229-5518
fading channels at the same decoding complexity. TTCM has shown superior performance over the other coded modulation schemes studied, but exhibited a higher error floor due to the uncoded information bits over uncorrelated narrowband Rayleigh fading channels[9].
As we have observed that the uniformly spaced constellations with equal probability for every signaling point shown an asymptotic gap of about 1.533dB (Shaping Gap) between the achievable performance of TCM and Channel capacity [10]-[13]. To narrow this gap, Gaussian signaling can be applied using shaping techniques, e.g., by assigning non uniform probabilities on different signaling points [13]-[17], the resulting advantage is referred as the shaping gain [14].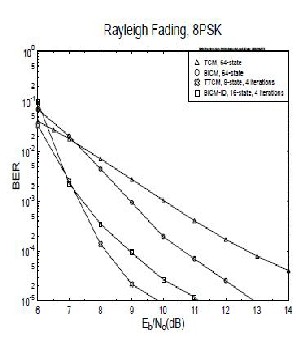
Figure 2: Comparison of TCM, TTCM, BICM and BICM-ID for 8PSK over uncorrelated Rayleigh fading channels using a block length of 4000 information bits.
Recently, superposition coded modulation (SCM) has been studied as an alternative approach to other bandwidth efficient coded modulation techniques [18],[19].With SCM, several coded sequences are linearly super imposed before transmission .When the number of layers are large, the transmitted signal exhibits an
Gaussian distribution which matches to an AWGN
channel. This provides a more straightforward method for achieving shaping gain [18], [20]. The work presented in [18]–[21] shows that such a concept is realizable with practical encoding and decoding methods. Simulation results show that an SCM scheme can operate within the shaping gap over AWGN channels [20], surpassing the theoretical limit of the uniform signaling based methods. In this paper, it will be shown that SCM also provides a simple and effective means of high-rate transmission over fading channels. By using low-rate component codes, significant diversity gains can be achieved with SCM. SCM also finds use in many other contexts, e.g., in the achievability proof of multi-user channel capacity in [22]– [24]. SCM has also been studied for practical broadcasting channel application, where it is shown that SCM can provide a significant gain over traditional time –division schemes. Another application of SCM is adaptive modulation through adjusting the number of layers and rate according to the channel condition[25].This is more flexible than traditional approaches,such as switching among different modulation schemes say,TCM using
8PSK(8-ary Phase shift keying), 16QAM(16-ary quadrature amplitude modulation),32-QAM,etc.,for channel adaption[26].The latter has the drawbacks of abrupt rate change and high receiver cost due to the need of many different TCM decoders.With SCM,change can be achived smoothly by using a low-rate code for each layer.The reciever cost can be kept low by using same code for all the layers and time –sharing a common decoder.
However, there is a practical limitfor SCM , the Gaussian-like transmitted signal has a relatively high peak- to-average power ratio(PAPR), which may cause a problem for radio frequency amplifier efficiency[27] the same PAPR problem also exists in other shaped coded modulation scheme,[14]–[17] and orthogonal frequency-division multiplexing (OFDM) systems. For OFDM systems, a number of PAPR reduction techniques have been studied (see [27] and references therein).Among these techniques, clipping is the most straightforward but may lead to substantial degradation in the bit-error-rate (BER) performance [27]–[36], especially for high-rate applications.
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 4
ISSN 2229-5518
We consider a K-layer SCM system. The encoding scheme is shown in Fig3. A binary data sequence u is partitioned into K subsequences {uk}. The kth subsequence uk is encoded by a binary encoder ( ENC-k) at the kth layer, resulting in a coded bit sequence Ck = {Ck ( j) } of length 2J, where Ck( j) € { 0,1 } and J is the frame length. The randomly interleaved version uk of Ck , from interleaver- k
(INTL-k), is then mapped to a quadrature phase shift
The clipped signal 
is then transmitted over a memory less channel. The received signal is given by
y(j) =h(j)
+w(j), j=0,1,….J-1 (5)
Where h(j) is the channel coeffeicient and w(j) is a complex,
keying (QPSK) sequence xk(j) = xkRe(j) + ixkIm(j)
zero-mean white Gaussian noise with variance 2
per
Figure 3: Encoder of superposition coded modulation system
Where i =
, the superscripts “Re” and “Im” are used to denote the real and imaginary parts of complex numbers , respectively, xkR e (j) = 1-2vk (2j) and xkR e (j) = 1-2vk (2j+1).
It is clear that xkR e (j) {+1,-1}and so does xkR e (j). The output signal at time j is a linear superposistion of K independently coded symbols
x(j) =
, j=0,1….J-1 (4)
Where {
k} are constant weighing factors. The overall rate is R=2
in bits/symbol, where Rk is the rate of the
kth binary component code.
dimension. The ratio of energy per bit (Eb) to the noise power spectral density (No= 2 2) is given by
Eb/No= E[ 
.
When K is large, x(j) can be approximated by a Gaussian random variable from the central limit theorem. Using Price’s theorem for nonlinear systems with Gaussian inputs [32], we can model the clipping operation as a linear process
(j) =
(6)
Where is a constant attenuation factor, and d(j) is a Gaussian distributed distortion term with zero mean and variance d 2 depend on 
and the statistics of x(j) , and can be calculated as [19][34]
= 
(7)
d 2 = (8) Where * denotes complex conjugate. Then the received
signal can be alternatively written as
y(j )=
(9)
Let E[.] denote the mathematical expectation and |.|
the amplitude .The PAPR of x(j) is defined as
PAPR =10 log10( ) (10)
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 5
ISSN 2229-5518
For an SCM scheme with {β0=…= βK-1}, the PAPR is K .In order to suppress PAPR, we can clip x(j) before transmission according to the following rule[48]:
= Ax(j)/|x(j)|, |x(j)|
(11) Where A>0 is the clipping threshold. We define the clipping
ratio (CR,
) in decibel as
10( ) (12)
For example, for 4-layer SCM with { 
=1,
k},the maximum PAPR =6.02dBis reached at { βk =0, k }.
It is interesting to compare SCM with other alternative coded modulation schemes. In the following, we will focus on comparison with BICM [40]-[46]. SCM and BICM are closely related. We denote v(j)={v(j), v(j) ,…. v(j)} where v(j) {0,1} is the mth coded bit carried by x(j). With BICM, the transmitted signal x(j) is generated using , instead of (1), a more general mapping rule:
x(j) = µ(v(j)) (13)
The image of µ (.) is usually a 2 –ary constellation of uniformly distributed signaling points, but the principle can be generalized to non-uniform constellations. With this view, some comments are in order.
SCM is a special case of BICM since (4) is a special case of (13). As such, SCM may not outperform optimized BICM. The SCM in Fig.
3 involves multiple encoders while a BICM scheme usually involves only one overall encoder. We have observed that the performance of SCM with multiple encoders is better than that of SCM with a single encoder. (For the latter case, the signal in K
layers is generated by interleaving and segmenting the outputs of a single encoder.)
For very long codes in AWGN channels, SCM
is as good as BICM, since SCM can achieve near-capacity performance. Later, in Fig. 5, we will show that for short codes, BICM does have advantages in certain cases.
With QPSK modulation at each layer, SCM
optimization only involves K = M/2 weighting factors{βk} . BICM optimization is a much more complicated issue involving 2M constellation points [47].
The detection complexity for SCM is O(M) while that for BICM is O(2M). Therefore SCM has a complexity advantage for large M.
As demonstrated in Fig.5, given a target rate,
we can achieve diversity gain in SCM by decreasing the rate of each layer (and increasing K accordingly). The design and detection complexities of SCM grow linearly with K. For BICM, we can increase diversity gain by using larger constellations or rotating the signal constellations [40] but the design and detection complexities of these methods increase very quickly.
As explained below, both SCM and BICM
suffer from the high PAPR problem when OFDM is involved. But SCM is more robust to clipping effect compared to BICM.
In the following, we present several comparison examples based on turbo and convolutional codes.
We first compare SCM and BICM over AWGN channels. We consider R=2 bits/symbol. The rate-1/2 turbo code [41] (23,35)8 is employed in both schemes. For the SCM, we set K =2{Bk} ={1,1,51};PAPR =2.83 dB; the number of iterations is 9 in the DECs and 2 between the DECs and ESE. For the BICM, the Gray mapping is applied to the 16- QAM constellation; PAPR = 2.55 dB; the number of iterations in the DEC is 18. The two schemes have nearly the same complexity. Clipping is not considered here since the PAPRs are not significant. The performance with J =
2048 and 32,768 is shown in Fig.4. The BICM performance
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 6
ISSN 2229-5518
is better when J is small while the SCM performance surpasses that of BICM for a large J. (Similar observations have been made for convolution codes based schemes over AWGN channels.) One reason for this is that the suboptimal GA detection is used in SCM while the optimal MAP demapping is used in BICM. High length for SCM is only 1/K of that of BICM at fixed J, which affects interleaving gain [40]. (However, when J is large, the impact of interleaver length becomes less significant.)
4.6 Comparison in Fading Channels
Fig.6 compares SCM with BICM over fading channels at rates R = 2, 3 and 5 bits/symbol. The same component code with S =4 as that in Fig. 4 is again used here for SCM. For comparison, there BICM schemes with iterative decoding (BICM-ID) reported in [40],[47] and [46]are also simulated.
For BICM –ID the (23,35)8 convolutional code is directly used for R=2, and punctured to rate ¾ and 5/6 (using the optimal punctured pattern in [42]) for R=3 and 5 , respectively Clipping (with 
is applied to SCM , but
used for BICM-ID since the related PAPRs are small.
Here we would like to point out that the performance provided in fig 6. Are based on the best schemes known to us, although it may be possible to improve the BICM-ID performance through further optimization. With real {βk} and the GA method, the detection complexity of SCM is about 6 real multiplications, 6 real additions, and a tanh(.)Operation per coded bit [27]. As a comparison, in BICM-ID schemes employing 2M comparisons , 3×2M real additions and 2M table look-ups per coded bit, which can be very high when M is large (e.g.,M=6 for the 64-QAM signaling). We can show that, taking into account the APP decoding cost and the numbers of iterations needed, the overall complexities of the two schemes are comparable.
We briefly discuss the channels with OFDM
modulation. Both SCM and BICM suffer from the high
PAPR problem in this case. We apply clipping with =3 dB
to the two schemes and compare their performance. The frame length J is set to 2048 and the number of subscribers N is set to 256,this means that each frame contains J/N=8
OFDM blocks. For simplicity, the channel gains over subscribers are assumed to be independent, Rayleigh- distributed [29]. The coding schemes are the same as those in fig 6. The operations related to the cyclic prefix of OFDM are ignored in our simulations.
We observe that the soft compensation strategy is not effective for BICM –ID with the SP and MSP mappings. A similar observation is made in [45] and an explanation is provided there for this observation using the EXIT chart technique. On the other hand, compensation techniques are more effective for BICM with Gray mapping, but the resultant performance is still not satisfactory. Based on this, we adopt the signal model given by [18] and treat the clipping distortion as an equivalent AWGN for clipped BICM-ID. The results are compared with SCM in fig 7.
For reference, we have also included in fig .7 the results for
Gray –mapped BICM with clipping (
=3dB) and SC.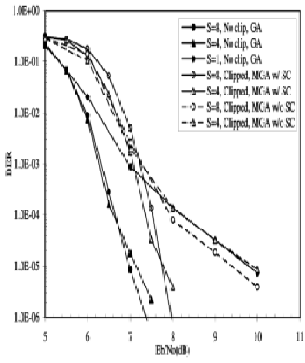
Figure 4: Performance of SCM at R=2 bits/symbol over fully interleaved Rayleigh fading channels, J=2048.The number
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 7
ISSN 2229-5518
of iterations is 10.For the clipped cases with SC, Qm=6 and
Qs=4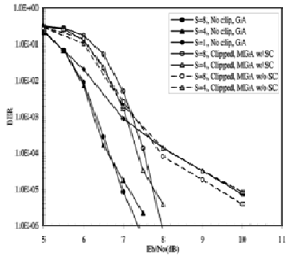
Figure 5: Performance of turbo coded ((23,35)8)SCM and
BICM at R=2 bits/symbol over AWGN channels.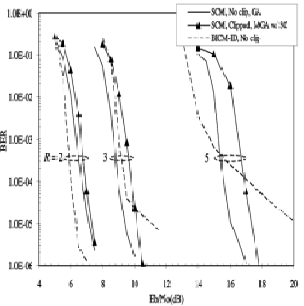
Figure 6: The performance of SCM and BICM-ID over fully
–inetrleaved Rayleigh fading channels, J=2048, for the SCM
without clipping , the number of iterations 12 for R=2 and
3, and 16 for R=5.For the clipped SCM,Qm=6 and Qs=6 For R=2 And 3,Qm=6 and Qs=10 for R=5 and number of iterations is 10 for all the BICM-ID results.
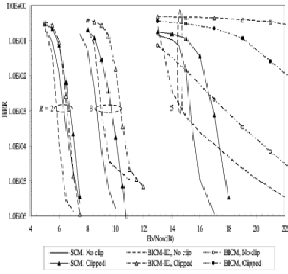
Figure 7: performance comparison of SCM and BICM-ID
with OFDM modulation over Rayleigh fading channels
;J=2048,N=256,The number of iterations are the same as those in fig for SCM and BICM-ID .For unclipped BICM,the number of iterations is 1 .For the clipped BICM Qm =1 and Qs=15.
We have compared SCM with BICM over various types of channels. It has been shown that, compared to BICM, SCM provides a simpler and more efficient means of achieving diversity gains for high–rate applications, especially when OFDM modulation with clipping is applied. The SCM performs better compared to BICM-ID for larger frame length and in multiuser environment for multiple access schemes.
The principles of Interleave Division Multiple Access can be extended from binary modulation to multi-ary modulation. Both SCM and IDMA can be combined with OFDM. Such combinations provide a solution to both multiple access interference (MAI) and inter-symbol interference (ISI) problems in multiple access schemes. It is also provides a solution to the peak-to-average-power-ratio (PAPR) problem in OFDM. The clipping distortion for peak power reduction can be minimized using a sub-optimal soft compensation technique. Several distinguished features of
the new scheme are
IJSER © 2013 http://www.ijser.org
Low PAPR,
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 8
ISSN 2229-5518
Robustness against fading,
Capacity achieving performance,
Flexibility in resource allocation,
The SCM –OFDM-IDMA is the future 4G technology for high data rate, which provides many desired features for modern communication systems, in particular, robustness against interference and both high power efficiency and spectral efficiency and it is a very flexible in allowing adaptive modulation and low-cost iterative detection in various channel condition .
[1] D. Divsalar and M. K. Simon, “The design of trellis coded MPSK for fading channel: Performance criteria," IEEE Transactions on Communications, vol. 36, pp. 1004-1012,
September 1988.
[2] G. Ungerboeck, “Channel coding with multilevel/Phase signal," IEEE Transactions on Information Theory, vol. 28, pp. 55-66, January 1982.
[3] D. Divsalar and M. K. Simon, “The design of trellis coded MPSK for fading channel: Set partitioning for optimum code design," IEEE Transactions on Communica- tions, vol. 36, pp. 1013-1021, September 1988.
[4] P. Robertson, T.W orz “Bandwidth-effecient Turbo Trellis- Coded Modulation Using Punctured Component Codes," IEEE Journal on Selected Areas in Communications, vol. 16, pp.
206-218, February 1998.
[5] C. Berrou, A. Glavieux and P. Thitimajshima, “Near Shannon Limit Error-Correcting Coding and Decoding : Turbo Codes," in Proceedings of IEEE International Conference on Communications, pp. 1064 - 1070, 1993.
[6] E. Zehavi, “8-PSK trellis codes for a Rayleigh fading channel," IEEE Transactions on Communications, vol. 40, pp. 873 { 883, May 1992.
[7] X. Li, J. A. Ritcey, “Trellis-Coded Modulation with Bit Interleaving and Iterative Decoding," IEEE Journal on Selected Areas in Communications, vol. 17, No. 4, April 1999.
[8] X. Li, J. A. Ritcey, “Bit-interleaved coded modulation withiterative decoding using soft feedback," IEEE Electronics Letters, vol. 34, No. 10, pp. 942 - 943, May 1998.
[9].S.X.Ng,T.H.liew,LL.Yang,L.Hanzo”Comparative study of TCM,TTCM,BICM and BICM-ID schemes”.
[10] G. D. Forney, Jr, “Trellis shaping,” IEEE Trans. Inf. Theory, vol. 38, pp. 281–300, Mar. 1992.
[11] R. F. H. Fischer, Precoding and Signal Shaping for Digital
Transmission. New York: Wiley, 2002.
[12] P. Limpaphayom and K. A. Winich, “Power- and bandwidth-efficient communications using LDPC codes,” IEEE Trans. Commun., vol. 52, pp. 350–354, Mar. 2004.
[13] N. Varnica, X. Ma, and A. Kavˇcic´, “Iteratively decodable codes for bridging the shaping gap in communication channels,” in Proc. Asilomar Conf. Signals, Syst., Comput., Pacific Grove, CA, Nov. 2002.
[14] L. Duan, B. Rimoldi, and R. Urbanke, “Approaching the AWGN channel capacity without active shaping,” in Proc. IEEE Int. Symp. Information Theory, Ulm, Germany, Jun./Jul. 1997, pp. 374–374.
[15] S. Gadkari and K. Rose, “Time-division versus superposition coded modulation schemes for unequal error protection,” IEEE Trans. Commun., vol. 47, pp. 370–379, Mar. 1999.
[16] X. Ma and L. Ping, “Coded modulation using superimposed binary codes,” IEEE Trans. Inf. Theory, vol.
50, pp. 3331–3343, Dec. 2004.
[17] X. Ma and L. Ping, “Power allocations for multilevel coding with sigma mapping,” Electron. Lett., vol. 40, no. 10, pp. 609–611, May 2004.
[18] T. M. Cover and J. M. Thomas, Elements of Information
Theory. New York: Wiley, 1991.
[19] D. N. C. Tse and P. Viswanath, Fundamentals of Wireless
Communication. Cambridge, U.K.: Cambridge Univ. Press,
2005.
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 9
ISSN 2229-5518
[20] P. Wang, J. Xiao, and L. Ping, “Comparison of orthogonal and nonorthogonal approaches to future wireless cellular systems,” IEEE Veh. Technol. Mag, vol. 1, no. 3, pp. 4–11, Sep. 2006.
[21] H. Schoeneich and P. A. Hoeher, “Adaptive interleave- division multiple access-A potential air interface for 4G bearer services and wireless LANs,” in Proc. 1st IEEE and
IFIP Int. Conf. Wireless and Opt. Commun. Netw. (WOCN
2004), Muscat, Oman, Jun. 2004, pp. 179–182.
[22] A. Goldsmith and S.-G. Chua, “Adaptive coded modulation for fading channels,” IEEE Trans. Commun., vol.
46, pp. 595–602, May 1998.
[23] S. H. Han and J. H. Lee, “An overview of peak-to- average power ratio reduction techniques for multicarrier transmission,” IEEE Wireless Commun., vol. 12, no. 2, pp.
56–65, Apr. 2005.
[24] X. Li and L. J. Cimini, “Effects of clipping and filtering on the performance of OFDM,” IEEE Commun. Lett., vol. 2, pp. 131–133, May 1998.
[25] H. Ochiai and H. Imai, “Performance analysis of deliberately clipped OFDM signals,” IEEE Trans. Commun., vol. 50, pp. 89–101, Jan. 1999.
[26] J. Armstrong, “Peak-to-average power reduction for OFDM by repeated clipping and frequency domain filtering,” Electron. Lett., vol. 38, pp. 246–247, Feb. 2002.
[27] J. Tellado, L. M. C. Hoo, and J. M. Cioffi, “Maximum- likelihood detection of nonlinearly distorted multicarrier symbols by iterative decoding,” IEEE Trans. Commun., vol.
51, pp. 218–228, Feb. 2003.
[28] A. R. S. Bahai, M. Singh, A. J. Goldsmith, and B. R. Saltzberg, “A new approach for evaluating clipping distortion in multicarrier systems,” IEEE J. Sel. Areas
Commun., vol. 20, pp. 1037–1046, Jun. 2002.
[29] D. Kim and G. L. Stüber, “Clipping noise mitigation for OFDM by decision-aided reconstruction,” IEEE Commun. Lett., vol. 3, pp. 4–6, Jan. 1999.
[30] H. Chen and A. M. Haimovich, “Iterative estimation and cancellation of clipping noise for OFDM signals,” IEEE Commun. Lett., vol. 7, pp. 305–307, Jul. 2003.
[31] M. Colas, G. Gelle, and D. Declercq, “Analysis of iterative receivers for clipped COFDM signaling based on soft turbo-DAR,” in Proc. Int. Symp. Wireless Commun. Syst.,
2004.
[32] H. Nikopour, A. K. Khandani, and S. H. Jamali, “Turbo-coded OFDM transmission over a nonlinear channel,” IEEE Trans. Veh. Technol., vol. 54, no. 4, pp. 1361–
1371, Jul. 2005.
[33] R. Price, “A useful theorem for nonlinear devices having gaussian inputs,” IRE Trans. Inf. Theory, vol. IT-4, pp. 69–72, Jun. 1958.
[34] L. H. Liu, J. Tong, and L. Ping, “Analysis and optimization of CDMA systems with chip-level interleavers,” IEEE J. Sel. Areas Commun., vol. 24, pp. 141–
150, Jan. 2006.
[35] L. Ping, L. H. Liu, K. Y. Wu, and W. K. Leung, “Interleave-division multiple-access,” IEEE Trans. Wireless Commun., vol. 5, no. 4, pp. 938–947, Apr. 2006.
[36] E. Zehavi, “8-PSK trellis codes for a Rayleigh channel,”
IEEE Trans. Commun., vol. 40, pp. 873–884, May 1992.
[37] G. Caire, G. Taricco, and E. Biglieri, “Bit-interleaved coded modulation,” IEEE Trans. Inf. Theory, vol. 44, pp.
927–946, May 1998.
[38] A. Chindapol and J. A. Ritcey, “Design, analysis, and performance evaluation for BICM-ID with square QAM constellations in Rayleigh fading channels,” IEEE J. Sel.
Areas Commun., vol. 19, pp. 944–957, May 2001.
[39] J. Tan and G. Stüber, “Analysis and design of symbol mappers for iteratively decoded BICM,” IEEE Trans. Wireless Commun., vol. 4, no. 2, pp. 662–672, Mar. 2005.
[40] N. H. Tran, H. H. Nguyen, and T. Le-Ngoc, “Performance of BICM-ID with signal space diversity,” IEEE Trans.Wireless Commun., vol. 6, no. 5, pp. 1732–1742,
May 2007.
IJSER © 2013 http://www.ijser.org
International Journal of Scientific & Engineering Research Volume 4, Issue 1, January-2013 10
ISSN 2229-5518
[41] S. X. Ng, T. H. Liew, and L. Hanzo, “Comparative study of TCM, TTCM, BICM and BICM-ID schemes,” in Proc. IEEE VTC’2001, Rhodes, Greece, May 2001, pp. 2450–
2454.
[42] S. t. Brink, J. Speidel, and R. Yan, “Iterative demapping and decoding for multilevel modulation,” in Proc. IEEE GLOBECOM, Nov. 1998, pp. 579–584.
[43] S. t. Brink, “Convergence behavior of iteratively decoded parallel concatenated codes,” IEEE Trans. Commun., vol. 49, pp. 1727–1737, Oct. 2001.
[44] S. ten Brink, “Rate one-half code for approaching the
Shannon limit by 0.1 dB,” Electron. Lett., vol. 36, no. 15, pp.
1293–1294, Jul. 2000.
[45] S. Benedetto and G. Montorsi, “Unveiling turbo codes: Some results on parallel concatenated coding schemes,” IEEE Trans. Inf. Theory, vol. 42, pp. 409–428, Mar. 1996.
[46] C. Berrou, A. Glavieux, and P. Thitimajshima, “Near Shannon limit error-correcting coding and decoding: Turbo-codes,” in Proc. IEEE Int. Conf. Commun., Geneva, Switzerland, May 1993, pp. 1064–1070.
[47] J. G. Proakis, Digital Communications, 4th ed ed. New York: Mc- Graw-Hill, 2000. Authorized licensed use limited to: CityU. Downloaded on September 23, 2009.
[48].Jung Tong,Li Ping,”Superposition coded
Modulationwith Peak-Power Limitation”,IEEE Transaction on Information Theory,Vol.55.No.6,June -2009.
IJSER © 2013 http://www.ijser.org