The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 1

ISSN 2229-5518

On Existence and Asymptotic Behavior of the Solution for

a Fractional Integro-Differential Equation Azhaar H. Sallo* Afrah S. Hasan* azhaarsallo@gmail.com afrahsadiq8@gmail.com March 8, 2012

*Department of Mathematics, Faculty of Science, University of Duhok, Kurdistan Region, Iraq.

Abstract

In this paper, we have taken a fractional integro-differential equation of Volterra nonlinear type integral equation with initial conditions, and we have studied the existence and asymptotic behavior

of solution in the space of p-integrable functions on (0,b ) ,

L p (0,b ), 1

p . Our method is based

on the applications of the Banach fixed point theorem, generalized Gronwall’s lemma and Hölder's
inequality.

Keywords: Asymptotic behavior of solution, Banach fixed point theorem, generalized Gronwall’s lemma and Hölder's inequality

1 Introduction

In this paper, we consider the existence and asymptotic behavior of solution of the following fractional integro-differential equation

I y (x )

f (x , y (x ))

g (x ,t , y (t ))dt ,0 x b , n 1

n , n 2,

(1.1)

with the initial conditions:

y (i ) (0)

l i 1

l i 1 R ,

for i
0,1, 2,...,n 1,
(1.2)
Where f

: 0,b R R and

g : 0,b

0,b R R are continuous functions, R is the set of real
numbers. Fractional integro-differential equations play an important role in modeling processes in

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 2

ISSN 2229-5518

applied mathematics in various fields (physics, engineering, finance, biology....). There are many real
life problems that can be modeled by fractional differential equations in acoustics, electromagnetic, diffusion processes, viscoelasticity, hydrology, heat conduction in materials with memory, and other areas, see [2, 5, 6] for more details.
Nowadays, fractional integro-differential equation has gained great authors' attention, because of its
wide range of applications. Some existence and asymptotic behavior of solutions of fractional integro- differential equations recent works considered by Momani S. et al [3], [4]. Wu J. et al [8], Ahmad B. et al [1].

2 Preliminaries

First, we shall set forth some preliminaries and hypotheses that will be used in our discussion, for
details see [2, 7].
Definition 2.1. . Let f be a function which is defined almost everywhere (a.e) on

a,b . For 0 ,we
define

x I f

1 x t

( )

1f (t )dt

provided that this integral (Lebsegue) exists, where is gamma function. where n

[ ] denote the integer part of .

[ ] 1 and

Definition 2.2. For a function f given on the interval[a, b ] , the Caputo fractional derivative of order

0 , of f is defined by

x 1 ( n ) n 1

Lemma 2.1. Let 0 , then:

a Ic f

(n ) a
(t ) x t dt

x t 2 n 1

a I a I c

f f (x ) c0

c1x c2x

...

cn 1x

for some ci

R , i=0, 1, 2, …, n-1, n

[ ] 1.

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 3

ISSN 2229-5518

Lemma 2.2. If 0 and f L (a, b ) , then x I f exists everywhere if 1
and (

a.e.) if 1. for
all x

[a, b ] .

Lemma 2.3. If 1 and f

(x )

L (a, b ) , then x I f is absolutely continuous in x
[a, b ] .

Lemma 2.4. (Hölder's inequality)

Let X be a measurable space, let p and q satisfy 1 p

, 1 q

, and

1 1 1. p q

If f

Lp ( X )

and g

Lq ( X ), then ( f g )

belongs to

L( X ) and satisfies

f g dx

1 p

f p dx

1 q

g q dx .

Lemma 2.5. (Generalized Gronwall's Lemma).

Let

w (x ) , v (x )

and

y (x ) be continuous functions in a subinterval of R . Assume that

y and v are continuous and w is a non-decreasing that its negative part is integrable on every closed

and bounded subinterval of . If y is non-negative and if v satisfies the integral inequality:

y( x)

w( x)

v(t) y(t) dt , for all x x 0

then

y( x)

w( x) exp(

v(t ) dt )

, for all x x 0 .
Theorem 2.1. If there exists a Lebesgue integrable function g on where f is measurable, then f Lebesgue integrable function.

3 The Main Results.

a,b such that f g a.e. on

a,b

In this section, we shall prove existence of solution in

L p (0,b ) , also we study the asymptotic

behavior of solutions in

L p (0,b )

as b , for the fractional integro-differential equation (1.1)
satisfying (1.2).

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 4

ISSN 2229-5518

Consider

L p (0,b ) to be the space of all measurable functions f such that

f is Lebesgue

b p p

integrable on (0,b ) . For any

f L p (0,b )

, we define the norm as

f f dx , under this

norm, the space

L p (0,b ) is a Banach space.

Lemma 3.1. Let n 1

n and

f : 0,b R  R

be continuous. A function y is a solution of
fractional integral equation

n 1 l x i 1 x

1 x t

y (x ) i 1

(x t )

1f (t , y (t ))dt

(x t )

1 g (t , s , y (s ))ds dt

(3.1)

i 0 i ! ( ) 0

( ) 0 0
if and only if it is a solution of a fractional integro-differential equation (1.1) satisfying (1.2).

Proof. Operate both sides of equation (1.1) by the operator x I we obtain

x x t x x

0 I 0 I y (x )

0 I f

(x , y (x ))

0 I g (x ,t , y (t )) dt

by using Lemma 2.1, we get

y (x )

c c x c x 2

 c x n 1

x I f

(x , y (x ))

x I g (x ,t , y (t )) dt

(3.2)

0 1 2

n 1 0 0

y (x ) c

2c x
 (n
1) c x n 2

x I 1f

(x , y (x ))

x I 1

g (x ,t , y (t )) dt

1 2 n 1 0 0

y (x ) 2c

 (n
1) (n
2) c x n 3

x I 2f

(x , y (x ))

x I 2

g (x ,t , y (t )) dt

2 n 1 0 0



y ( n 1) (x ) (n

1) (n
2) (n
3)...2 c

x I n f (x , y (x ))

x I n

g (x ,t , y (t )) dt

n 1 0 0

0 ,

using the initial conditions (1.2), we obtain

c l , c

1 l , c

1 l ,c

1 l , ...,c 1 l

(3.3)

0 1 1

1! 2 2

2! 3 3

3! 4

n 1 (n

1)! n

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 5

ISSN 2229-5518

substituting equation (3.3) in equation (3.2), we obtain the final form of

y (x ) as equation (3.1).

Theorem 3.1: Let the right hand side of the fractional integro-differential equation (1.1) satisfy the

following conditions:

i- f (x , y (x )) ,

g (x ,t , y (t )) dt Lp

0,b ,
ii-

f (x , y 2 (x ))

f (x , y 1 (x ))

Z (x ) y 2

y 1 and

g x ,t , y 2 (t )

g (x ,t , y 1 (t ) dt P (x ) y 2 y 1

for all x

(0,b ) and

y 1 , y 2

Lp 0,b , where

Z (x )

and

P (x )

are non – negative continuous and
bounded functions on 0,b , then if:

2 b k 1 k 2 p

p p 1 1

(3.4)

p p 1

there exists a p–integrable solution of the fractional integro-differential equation (1.1) satisfying (1.2).
Proof. Let the mapping T on Lp
0,b be defined as

n 1 l x i 1 x

1 x t

T y (x ) i 1

(x t )

1f (t , y (t )) dt

(x t )

1 g (t , s , y (s )) ds dt (3.5)

i 0 i ! ( ) 0

( ) 0 0
we have to prove that T maps Lp
0,b into itself. Let:

n 1 l x i 1 x

1 x t

h (x ) i 1

(x t )

1f (t , y (t )) dt

(x t )

1 g (t , s , y (s )) ds dt

i 0 i ! ( ) 0

n i

( ) 0 0

x x t p

p 1 l x 1 1

h (x ) i 1

(x t )

1f (t , y (t )) dt

(x t )

1 g (t , s , y (s )) ds dt

i 0 i ! ( ) 0

( ) 0 0

p n 1 l xi

2 p x

p p 2 p x t

h ( x) 2 p i 1

( x t )

1 f (t, y(t)) dt

( x t)

1 g (t, s, y(s)) ds dt

p p

i 0 i ! ( ) 0

( ) 0 0

n 1 l x i x x t

let

h1 (x )

i 1

i 0 i !

, h (x ) (x t ) 1

f (t , y (t )) dt

and h (x ) (x t ) 1

0 0

g (t , s , y (s )) ds dt .

h1 (x )

is continuous , thus it is measurable, hence

h1 (x )

is measurable and it is Lebesgue integrable.

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 6

ISSN 2229-5518

Now we take

h2 (x )

(x t )

1f (t , y (t )) dt (3.6)

by Lemma 2.2 and Lemma 2.3

h2 (x )

exists and it is absolutely continuous and so it is continuous.
Thus

h2 (x )

is measurable and hence

h2 (x )

is measurable. Then we must show that

h2 (x ) is

Lebesgue integrable. Since by condition (i)

f ( x, y( x))

Lp (0, b)

and we have

(x t) 1

Lq (0, b) , then by

Hölder's inequality and from equation (3.6) we obtain

1 1 p

x q x p

h2 (x )

(x t )q

1 dt f

(t , y (t )) dt

0 0

x p 1

p 1

1 1 x

1 1

(t , y (t )) dt

x p 1

p 1

f (t , y (t )) dt

p 1 0

from definition (2.1), for 0 x b , we have:

x t

f (t , y (t ))

0 0

ds dt =

x t f

(t , y (t ))

dt =

x I 2 f (t , y (t )) p .

Thus by Lemma 2.2, it follows that

f (t , y (t ))

dt is Lebesgue integrable for all x

(0,b ) , hence by

Theorem 2.1

h2 (x )

is Lebesgue integrable. Now we take

x t p

h3 (x )

(x t ) 1

g (t , s , y (s )) ds dt (3.7)

0 0

By condition (i),

g (x ,t , y (t )) dt L p o

0,b , and we have

(x t) 1

Lq (0, b)

then by Hölder's inequality
and from equation (3.7) we obtain

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 7

ISSN 2229-5518

1 1 p x q x t p p

h3 ( x)

( x t )q

1 dt g (t, s, y(s))

ds dt

0 0 0

x p 1

p 1

x t p

g (t , s , y (s )) ds dt

p 1 0 0

from definition (2.1), for 0 x b , we have:

x t s

0 0 0

g (s , , y ( ))

d ds dt

x t

x t g (t , s , y (s ))

0 0

ds dt

t p x 2 g (t , s , y (s )) ds

thus by Lemma 2.2 , it follows that

x t

g (t, s, y(s))

0 0

ds dt is Lebesgue integrable for all x

(0,b )

and by Theorem 2.1 we have

h3 (x )

is Lebesgue integrable, so

h (x )

is Lebesgue integrable,
therefore T maps

L p (0,b )

into itself. Now, to prove that T is a contraction mapping on

L p (0,b ) , let

y 1, y 2

Lp (0,b ) then

T y T y

1 x t

f t , y

(t )

f t , y

(t ) dt

2 1 2 1

x t p

1 x t

g t , s , y

(s )

g t , s , y

(s )

ds dt

2 1

0 0

2p b x

x t

f t , y

(t )

f t , y

(t ) dt

p 2 1

0 0

x t

x t g t , s , y 2 (s )

0 0

g t , s , y 1 (s )

ds dt dx

by using condition (ii) we get

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 8

ISSN 2229-5518

p b x

p x p

Ty Ty 2

x t Z (t )

y y dt x t

P (t )

y y dt dx by

2 1 p

2 1 2 1

0 0 0

Hölder's inequality

b x

p q 1

1 p

1 p q x

p ( ) p

T y 2

T y 1

p x t dt Z t y 2

0 0 0

y 1 dt dx

1 1 p

2p b x

q 1 q

x p

p ( ) p

+ p x t dt P t y 2

0 0 0

y 1 dt dx

p 1 p b x

( p 1) 2

p 1 p

x p 1

Z p (t ) y y

dt dx

( p 1) 0 0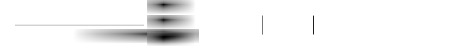

b x

x p 1

P p (t ) y y

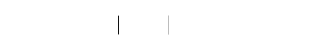
dt dx (3.8)

0 0

to evaluate the integral in the right hand side of inequality (3.8) , let

r (x )

Z (t ) y 2

y 1 dt

r (x )

Z (x ) y 2 y 1

w (x )

P (t ) y 2

y 1 dt

w (x )

P (x ) y 2 y 1

hence inequality (3.8) becomes

p 1 p b b

Ty 2 Ty 1

( p 1) 2

p 1 p

x p 1 r (x )

dx x p

1 w (x ) dx

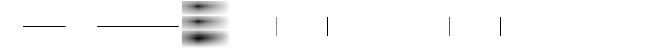
( p 1) 0 0

p 1 p b b

p 1 2

b p Z p (x ) y y

dx x p

Z p (x ) y y dx

p 1 p

p 2 1 2 1

0 0

b b

b p P p

(x ) y 2

y dx x p P p

(x ) y 2

y 1 dx .

(3.9)

0 0

Since

x p Z p

(x ) y 2

y 1 dx

0 and

x p P p

(x ) y 2

y 1 dx

0 , hence inequality (3.9) becomes

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 9

ISSN 2229-5518

p 1 p b b

Ty Ty

p 1 2

b p z p (t ) y y

dt b p

P p (t ) y y dt

2 1 p 1 p

p 2 1 2 1

0 0

since

z (x ) and P (x ) are non-negative continuous and bounded functions , therefore

z (x )

k 1 , P (x ) k 2

for all x

0,b , so

p 1 p p p p b

p 1

Ty 2 Ty 1 p 1

2 b k 1 k 2

y 2 y 1 dt

p 1 p p p b

p 1

Ty 2 Ty 1 p 1

2 b (K 1

K 2 )

y 2 y 1 dt

2b (K 1 K 2 ) p 1 .

Ty Ty y y

2 1 p 1 2 1

p p 1

From (3.4), T is a contraction mapping on

L p (0,b ) . Thus T has a fixed point say

y (x )

L p (0,b )

that

is Ty (x )

y (x ) .

Next, we study the asymptotic behavior of solutions for the fractional integro-differential
equation (1.1) satisfying (1.2).

Theorem 3.2. Let the functions

f ( x, y)

and

g ( x, t, y(t ))

of the fractional integro-differential
equation (1.1), satisfy the following conditions:

f (x , y (x )) (x )

y (x )

(3.10)

g (x ,t , y (t )) dt

(x )

y (x )

(3.11)

where (x ) and (x ) are continuous functions for all x

(0,b ) as b , such that

lim x t

1 (x ) (x )

dt M

, M 0

(3.12)

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 10

ISSN 2229-5518

then

y (x )

, where

y (x ) is a solution of fractional integro-differential equation (1.1) satisfying (1.2),

n 1 l x i

is asymptotic to K i 1

i 0 i !

as x tends to infinity.

Proof. Any solution of the fractional differential equation (1.1) satisfying (1.2) is defined by

n 1 l x i 1 x

1 x t

y (x ) i 1

(x t )

1f (t , y (t ))dt

(x t )

1 g (t , s , y (s )) ds dt

i 0 i ! ( ) 0

( ) 0 0

n 1 l x i 1 x

1 x t

y (x ) i 1

(x t )

1 f (t , y (t )) dt

(x t ) 1

g (t , s , y (s )) ds dt

i 0 i ! ( ) 0

( ) 0 0
by using conditions (3.10) and (3.11), we get:

x x

y (x )

H (x )

1
( ) 0
(x t ) 1
(t )

y (t ) dt

1
( ) 0
(x t ) 1
(t )

y (t ) dt (3.13)

n 1 l x i

where

H (x )

i 1

i 0 i !

is a non-decreasing function. From inequality (3.13) and Gronwall's Lamma
and by using condition (3.12), we obtain:

y (x )

H (x ) exp

1 (x t ) 1
(t ) (t ) dt

y (x )

( ) 0

H (x ) exp M 1

where M 1

( ) , M 1 is a positive constant, thus

y (x )

K H (x )

for all x 0

, K exp(M 1 ) , we have

n 1 l x i

y (x )

K i 1 .

i 0 i !

So y (x )

has the given asymptotic property, hence the proof is complete.
The following example is an application of the theorem (3.2).

Example. Consider the following fractional integro-differential equation

y (3.25) (x )

e 2x y (x )

1 cosh(x )

(e t

te t ) y (t ) dt

, 3 3.25 4 , (3.14)

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 11

ISSN 2229-5518

with initial conditions:

y (0) 1,

y (0) 2 , y

(0) 3 and y

(0) 4 . (3.15)

Here we have

f (x , y (x ))

e 2 x

y (x )

and

g (x ,t , y (t ))

(e t

te t ) y (t )

1 cosh(x )

where f (x , y (x ))
and

g (x ,t , y (t )) satisfy the conditions (3.10) and (3.11) as follows

e 2x

y (x )

e y (x )

1 cosh(x )

Moreover

(e t

te t ) y (t ) dt

x e x y (x ) , (using the integration by parts).

(t x )2.25 ( e

te t )dt

1 t 2.25e

t dt t 3.25e

t dt

1 t 2.25e

t dt t 3.25e

t dt ,

x 1 cosh(t ) 2 x x
2 0 0
by the definition of Gamma function we get
(t x )2.25 ( e

te t )dt

1 (3.25) (4.25) 9.55968 ,

x 1 cosh(t ) 2
therefore by Theorem 3.2 the solution of the fractional integro-differentia equation (3.14) satisfying

3x 2

4x 3

(3.15) is asymptotic to

H (x )e 4.4229 , where

H (x ) 1 2x .

2 6

References

[1] Ahmad B., J. J. Nieto; Existence results for nonlinear boundary value problems of fractional integrodifferential equations with integral boundary conditions, Boundary Value Problems, 2009,
dio:10.1155/2009/708576.

The research paper published by IJSER journal is about On Existence and Asymptotic Behavior of the Solution for a Fractional Integro-Differential Equation 12

ISSN 2229-5518

[2] Mainardi F., Fractional calculus: ’Some basic problems in continuum and statistical mechanics’, In

fractals and fractional calculus in continuum mechanics, A. Carpinteri and F. Mainardi (eds.), Springer-Verlag, New York, 1997, 291-348.

[3] Momani S. and Hadid S.B., Lyapunov stability solutions of fractional integrodifferential

equations, J. Fract. Calc., 18 (2003).

[4] Momani S., Jameel A.and Al-Azawi S., local and global uniqueness heorems on fractional integro-differential equations via Bihari’s and Gronwall’s inequalities, Soochow Journal of Mathematics, Vol. 33(2007), No. 4, pp. 619-627.

[5] Podlubny, Fractional Differential Equations, Academic Press, New York, 1999.

[6] Riu D.and Reti´ere N., Implicit half-order systems utilisation for diffusion phenomenon modelling, In Fractional Differentiation and its Applications, Eds. A. Le Mahaute, J.A. Tenreiro Machado, J.C. Trigeassou and J. Sabatier, Ubooks Verlag, Neusb, 2006, 447-459.

[7] Samko S.G., Kilbas A.A. and Marichev O.I., Fractional Integrals and Derivatives; Theory and

Applications. Gordon and Breach, Amsterdam 1993.

[8] Wu J., Liu Y., Existence and uniqueness of solutions for the Fractional integro -differential equations in Banach spaces, Electronic Journal of Differential Equations, Vol. 2009(2009), No. 129, pp.
1–8.